
As we can see, g-loads required to pull out of a dive from the top of the VDOT antenna are impossible for a 757.
-- Rob Balsamo, co-founder of Pilots for 9/11 Truth, narrating the video 9/11: Attack on the Pentagon
It is possible to prove a negative.
Freshman-level Newtonian mechanics can give us a lower bound for the g-load that an aircraft will experience when pulling out of a dive.
If all plausible flight paths over the top of the VDOT antenna that end in level flight at the Pentagon involve g-loads that vastly exceed the capabilities of a Boeing 757, then American Airlines Flight 77 did not fly over the top of the VDOT and end in level flight at the Pentagon on 11 September 2001.
Rob Balsamo calculated that all such flight paths would involve at least 11.2 g, but made several serious mistakes in that calculation. A week later, Balsamo admitted his errors and promised to "publish a revision with the proper formula(s)/calculations".
9/11: Attack on the Pentagon is that promised revision, in the form of a 50-minute video. In that video, Balsamo recalculated the g-load using a completely different method, coming up with a lower bound of 10.14 g.
As shown below, however, there exists a flight path that goes over the top of the VDOT tower, ends in level flight at the Pentagon, and requires less than 2 g.
For more plausible flight paths that pass beside the VDOT tower instead of over it, the g-load is about 1.6 g.
This is not a matter of "he said, she said". It is a matter of mathematics and physics. Once the problem and its parameters have been set, there is one correct answer and many wrong answers.
In this review of 9/11: Attack on the Pentagon, I will explain how Rob Balsamo came up with his wrong answers. I will also explain several different correct ways to perform the calculation.
There is alot of math required to understand this so you might consult Larry the math wizard.
http://vodpod.com/watch/1632533-911-attack-on-the-pentagon-- Michael Thames, 11 September 2009, posted to
rec.music.classical.guitar
This review will focus on mathematics and physics.
First, however, I will explain how this elementary problem in Newtonian mechanics came to my attention, and why this calculation is so important for Rob Balsamo and the no-757-at-the-Pentagon fringe of the 9/11 truth movement.
Then I will identify the mistakes in Balsamo's original calculation, and show how to fix them. We will then calculate correct answers for several variations of the problem.
I will then reformulate the problem as a 2-dimensional boundary value problem, and find a general solution to that 2-dimensional problem. We will then calculate correct answers for the 2-dimensional problem. Those answers will be the same answers we had calculated for the original 1-dimensional problem. That is not just a coincidence.
I will then explain several of the mistakes made in Rob Balsamo's video. In particular, I will explain how Balsamo's curve-fitting technique allows a range of incorrect answers to be calculated, from which a calculating practitioner of the devious arts might select whatever incorrect answer is desired.
Message from Rob;
lol... i wouldnt even attempt to remember my login at that place.
Just keep asking Clinger why he spends all his time obsessed with me and if so obsessed, why doesnt he just email us for a proper debate?
The reason why he will never do this, is because he knows he is wrong. Clinger remains behind his screen where he belongs.
-- Michael Thames, 15 September 2009, posted to
rec.music.classical.guitar
Michael Thames is a guitar-maker who lives near Sante Fe, New Mexico.
In the message above, Thames wanted us to think he was quoting Rob Balsamo. It certainly sounds like Balsamo. But was it?
In the past, when pressed, Michael Thames has buttressed his arguments by quoting unnamed friends who (according to Thames) work at one of the national laboratories in New Mexico. According to Thames, one of those unnamed friends told Thames that the "top physicist" at Sandia National Laboratories "fervently believes" the moon landings were faked. Should we believe this? Does Thames even believe this? Hard to tell.
Michael Thames is an opinionated, passionate, and self-confident guy who fervently believes faces on Mars were created by an ancient Martian civilization, that the Apollo 11 astronauts saw a UFO on the moon (if they went to the moon at all), that a man who calls himself Dr Jonathan Reed captured an extra-terrestrial creature in 1996, that humans have walked the earth for hundreds of millions of years after devolving from pure spirit, that evolution is pseudoscience, that the Bush administration planned and executed the attacks of 11 September 2001, and that the Pentagon was not hit by American Airlines Flight 77 or by any other airliner.
Many people believe those things, and who's to say they're wrong? Of course, most people keep such beliefs to themselves when participating in a newsgroup devoted to the classical guitar. Not Michael. And so it was that Michael Thames urged classical guitarists worldwide to watch Rob Balsamo's video telling us why American Airlines Flight 77 couldn't possibly have crashed into the Pentagon on 11 September 2001.
That's how I learned of the video. Having experienced Rob Balsamo's June 2008 invasion of the classical guitar newsgroup, I wondered what Rob had been up to since.
And you didnt "drag" me into this my friend. I have some free time while rendering scenes for our latest production and i enjoy showing the public how some people go out of their way to make excuses.
-- Rob Balsamo, 25 June 2008, posted to
rec.music.classical.guitar
So I watched the video. Should you? If you already know that the US government planned and executed the attacks of 11 September 2001, then watching Balsamo's video may increase your smugness quotient. If you think the Truthers are as nutty as the Birthers, then watching the video may leave you shaking your head. If you approach the video with an open mind, you'll probably just fall asleep.
As a trained mathematician, however, I appreciated the video's creative use of mathematical techniques to reach a preconceived incorrect conclusion, together with the chutzpah required to dismiss the opposition's correct calculations using technical-sounding mumbo jumbo that most viewers won't have the background to evaluate.
The purpose of this review is to extend my appreciation of the video to a wider audience. Anyone who knows a little calculus and has taken a first course in Newtonian physics should be able to confirm my calculations. Readers with little or no technical background should still be able to understand the major problems with Balsamo's argument.
I love exposing these idiots. No worries MT... im having fun. :-)
[....]
MT, the people here are way behind the times with their arguments.. .and WAY out of their league. Stick around folks.. .watch them go down in flames... :-)-- Rob Balsamo, 25 June 2008, posted to
rec.music.classical.guitar
9/11: Attack on the Pentagon is a collaboration between Pilots for 9/11 Truth and Citizen Investigation Team (CIT). Pilots for 9/11 Truth is run by Rob Balsamo, who narrates the first part of the video. CIT consists of two people, Craig Ranke and Aldo Marquis, who contributed the second part of the video.
I really hate getting roped into this internet debates and prefer to do it over a recorded debate.
-- Rob Balsamo, 29 June 2008, posted to
rec.music.classical.guitar
Both Pilots for 9/11 Truth and CIT have annoyed some mainstream leaders of the 9/11 truth movement, who describe the entire no-757-at-the-Pentagon theory as a "booby trap" that "strains credulity and logic" and plays into the hands of those who wish to characterize the 9/11 truth movement as a collection of "lunatic conspiracy theorists". Their words, not mine.
Others have argued that the flight data recorder must be missing its final seconds due to the fact it shows too high and not enough gees to pull out of such a dive.
-- Rob Balsamo, 9/11: Attack on the Pentagon, 13:22-13:30
Contrary to Balsamo's statement above, the missing seconds are implied by the flight data recorder's own positional data, which end well short of the Pentagon, about twice as far as the Navy annex and VDOT antenna. The missing seconds have been confirmed by correlating the FDR's positional and altitude data with independent data obtained from ground radar and other sources. That correlation was performed by Rob Balsamo's former collaborator, engineer John Farmer (who should not be confused with attorney John J Farmer, Jr, senior counsel to the 9/11 Commission). Farmer's most important conclusion: "The FDR file positional data ends 6 ± 2 seconds prior to the reported impact location."
The NTSB flight path study for Flight 77 stops short of the Pentagon as well, but the NTSB also released a flawed animation of the final approach. The NTSB later described that animation as merely a "working copy" that was "never used for an official purpose", but the NTSB animation continues to serve as a straw man in 9/11: Attack on the Pentagon. An engineer named Mike Wilson has put together a much better animation of the approximate line of approach, matching the animation to photographs of the physical evidence, but Wilson's animation is not keyed to any data from the flight data recorder.
The controversy over how many of the flight data recorder's last few seconds of data are missing or corrupted undercuts most attempts to reconstruct the final approach from that data. That is why part 1 of the video and all of this review focus on a calculation that involves nothing more than
For more extensive discussion of the pertinent evidence and conspiracy theories involving Flight 77, readers should consult other sources.
After publishing the article showing that this hypothetical scenario would be impossible based on the government story and data, Lt Col Jeff Latas, core member of Pilots for 911 Truth, alerted us that the calculations were not accurate based on the premise of the original article.
-- Rob Balsamo, 9/11: Attack on the Pentagon, 2:56-3:15
Balsamo's original calculation was based on genuine numbers and would have yielded correct results if not for Balsamo's mistakes. That's more than can be said for the "corrected" calculation in 9/11: Attack on the Pentagon, so we will start by noting and correcting the errors in Balsamo's original calculation.
The original calculation begins with several geographical observations:
Balsamo believes the government's official flight path places the aircraft directly over the VDOT antenna, and supports his belief with a picture provided by CIT. In reality, several eyewitnesses have said the aircraft flew over the Navy annex or the road that lies between the Navy annex and the VDOT antenna. For future reference, let us note that the elevation of the Navy annex is about the same as the base of the VDOT antenna (135 feet above sea level) and that the Navy annex is a five-story building.
So far, the only implausible element of Balsamo's calculation is his insistence upon a flight path directly over the tallest obstacle in the area. All that remains is to calculate lower bounds for the g-load required:
At 781 ft/sec, the first 2400 horizontal feet would be covered in 3.07 sec, which Balsamo rounds down to 3 seconds. The last 1016 feet would be covered in 1.3 seconds.
The 224-foot drop from 304 to 80 feet therefore occurs in about 3 seconds, and the 47-foot drop from 80 feet to 33 feet occurs in about 1.3 seconds. The average rate of descent during the 224-foot drop is about 75 ft/sec, and the average rate of descent during the 47-foot drop is about 36 ft/sec.
Note that the 36 ft/sec figure results from Balsamo's assumption that the plane flew just over the top of pole 1. In reality, the plane struck pole 1, so the actual drop from pole 1 to the Pentagon was less than 47 feet, and the average rate of descent was less than 36 ft/sec.
For no reason at all, Balsamo assumes the instantaneous rate of descent at pole 1 is the same as the average rate of descent for the previous 3 seconds. That is implausible, because the plane should be leveling out at the end of those three seconds in preparation for descending at an average rate of less than 36 ft/sec over the final 1.3 seconds.
Balsamo can argue for whatever flight path he wants, but he can't choose one particular (highly implausible) flight path and then argue that the g-loads computed for his particular flight path are the lowest for all possible flight paths. To calculate a lower bound for g-loads, he must consider the flight path(s) whose g-loads are smallest. He doesn't even try to do that.
Having decided, however implausibly, that the rate of descent is 75 ft/sec at pole 1, he then multiplies 75 ft/sec by 1.3 seconds to get 97.5 feet. That is the vertical distance the plane would descend during the last 1.3 seconds if its rate of descent were 75 ft/sec throughout those 1.3 seconds. That would have put the plane more than 40 feet underground by the time it reached the Pentagon, which did not happen.
Balsamo then resorts to mathematical nonsense: He pretends his calculated 97.5 feet of distance is the same as an acceleration of 97.5 ft/sec2. He then divides 97.5 ft/sec2 by 32 ft/sec2 to get 3 g, and adds 1 g for gravity to get 4 g.
The guy doesn't even know how to check his units.
However, 97.5 feet vertically is not available.
-- Rob Balsamo, Arlington Topography, Obstacles Make American 77 Final Leg Impossible (emphasis in the original)
Balsamo then goes back to treating his bogus 97.5 ft/sec2 as a distance, 97.5 feet. For reasons that he explains (but do not make sense), he then reduces the 47-foot drop to a 35-foot drop. Because Balsamo will use the 35 feet as a divisor, this nonsensical adjustment increased the g-load as calculated by Balsamo.
Finally, Balsamo divides 97.5 feet (or is it 97.5 ft/sec2 ?) by 35 feet to obtain the dimensionless number 2.8 (or is it 2.8 per second squared?), and for no reason at all multiplies his 4 g by this meaningless number 2.8 to obtain 11.2 g.
Once again, Balsamo doesn't know how to check his units. And yet, in the video, you will hear Balsamo sneering at engineers, scientists, and mathematicians who actually know what they're doing and have performed the calculation correctly.
Having calculated a g-load of 11.2 g by making at least five serious mistakes, Balsamo notes that 11.2 g would "rip the aircraft apart", and concludes (incorrectly) that it is "impossible for any transport category aircraft to descend from top of VDOT to top of pole 1 and pull level" at the Pentagon.
The easiest way to refute Balsamo's conclusion is to perform the calculation correctly, and to provide mathematical descriptions of specific flight paths whose g-loads are well within the capabilities of a Boeing 757.
We will now
We will also consider a variation of the 2-dimensional problem in which the airspeed is held constant (instead of the ground speed), and obtain a solution to that variation of the problem in which the flight path is an arc of a circle instead of a segment of a parabola. We will find that the g-load for the circular solution is only slightly different than for the parabolic solution.
The reason for going through these mathematical exercises so carefully is that they will refute virtually all of the mathematical-sounding gibberish that Rob Balsamo intones during the video known as 9/11: Attack on the Pentagon.
Balsamo's original calculation assumed that the ground speed is a constant 781 ft/sec, and that is a useful simplifying assumption. With constant ground speed, all of the g-load comes from vertical deceleration and from gravity, so we can solve the problem by considering only the vertical distance, velocity, deceleration, and time to impact.
First we need a clear statement of the problem:
Calculate an achievable lower bound for the g-loads incurred by all flight paths that start at a point ‹ x1, y1 › near the Navy annex, maintain a constant ground speed of 781 ft/sec, and end in level flight at the Pentagon.
The laws of Newtonian mechanics are invariant under rigid transformations, so we get to choose a coordinate system that simplifies our calculations. I will take the base of the Pentagon as our origin ‹ 0, 0 ›, and measure elevations in feet above the base of the Pentagon. I will place the starting point (at the VDOT antenna or Navy annex) in the negative direction on the x axis. The flight path will begin at a negative time and end at the origin at time 0.
We will consider three flight paths:
For each of these flight paths, 3416 feet is the distance from the base of the VDOT antenna to the Pentagon. At 781 ft/sec, that distance will be covered in about 4.4 seconds. So the problem reduces to one of finding the vertical deceleration required to cover the vertical distance (whether 271, 180, or 120 feet) in 4.4 seconds and end up with a vertical velocity of zero (as required for level flight).
The minimal g-load is incurred by a flight path whose vertical deceleration is constant. For a constant acceleration or deceleration a, the distance covered in time t is
s = 1/2 a t2
Solving for the acceleration, we get
a = 2 s / t2
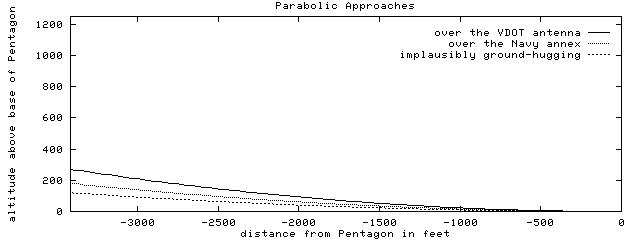
To convert that acceleration from ft/sec2 to g, we divide by 32 ft/sec2 and add 1 g for gravity. The following table shows the calculated vertical deceleration and g-load for each of our three flight paths.
| flight path | deceleration | g-load |
|---|---|---|
| over the VDOT antenna | 28.3 ft/sec2 | 1.9 g |
| over the Navy annex | 18.8 ft/sec2 | 1.6 g |
| implausibly ground-hugging | 12.5 ft/sec2 | 1.4 g |
Although 1.9 g would not be comfortable for passengers, it is within the limits of a Boeing 757. We cannot check these g forces against Flight 77's flight data recorder, because its positional data apparently end before the VDOT antenna, but the flight data recorder does record brief loads of up to 1.75 g before it reached the VDOT antenna.
If the 1-dimensional calculation above seems too simple, we can make it more complicated by formulating it as a set of ordinary differential equations. While we're at it, we'll convert it into a 2-dimensional boundary value problem.
Let f(t) be the position of the aircraft at time t. The aircraft must be sufficiently high at the VDOT antenna or Navy annex, it must collide with the base of the Pentagon at time 0, and its vertical velocity at impact must be 0 (for level flight). In addition, its horizontal velocity at impact is v0 = 781 ft/sec. The equations that f(t) must satisfy are:
To obtain a solution f(t) with constant ground speed and constant vertical deceleration a, we add the equation below. Specifying a zero horizontal acceleration implies a constant horizontal velocity (ground speed), so the time at which the aircraft is at the VDOT antenna or Navy annex is t1 = x1 / v0.
The solution to that boundary value problem is
f(t) = ‹ v0 t, 1/2 a t2 ›
where a = 2 y1 / t12. The first derivative of f is the velocity as a function of t, and the second derivative of f is the acceleration as a function of t:
f ′(t) = ‹ v0, a t ›
f ″(t) = ‹ 0, a ›
To check that f(t) as defined above is a solution, we just have to check each of the four equations:
The second derivative f ″(t) gives the acceleration, which is 0 in the horizontal direction and the constant value a = 2 y1 / t12 in the vertical direction. That's exactly the same as we had calculated for the simpler 1-dimensional problem, except we've renamed the vertical distance from s to y1. The g-loads for the various flight paths will therefore be exactly the same as was calculated for the 1-dimensional problem, and there is no need to repeat that table here.
Graphing y (elevation above the base of the Pentagon) versus x (distance from the Pentagon) for all three approaches, we get:
Heretofore we have assumed the ground speed is constant, as in Balsamo's original calculation. That isn't a bad approximation, because the vertical component of velocity is never more than 16% of the ground speed, but someone might reasonably insist that we consider solutions in which the air speed is held constant instead of the ground speed.
That changes the boundary value problem somewhat, so we will get slightly different results. The first three equations remain the same, but the fourth equation is replaced by
where v0 is the air speed, t1 is the time at the VDOT antenna or Navy annex (which must be calculated differently than before), and ¦ f ′(t) ¦ is the scalar velocity at time t.
The simplest solution for this new boundary value problem is a flight path that describes an arc of a circle instead of a segment of a parabola. The center of the circle will be a point ‹ 0, r › on the y-axis, where r is the radius of the circle. The equation for the full circle will be
r2 = x2 + (r - y)2 = r2 + x2 + y2 - 2 r y
For nonzero y, we can simplify that equation to
r = (x2 + y2) / (2 y)
Those equations already imply that the origin lies on the circle and that the flight path will be level at the origin. To calculate r, we use the fact that the flight path must go through the point ‹ x1, y1 ›, hence
r = (x12 + y12) / (2 y1)
For future reference, let's calculate the radius r for each of the three flight paths we're considering.
| flight path | x1 | y1 | r |
|---|---|---|---|
| over the VDOT antenna | -3416 ft | 271 ft | 21665 ft |
| over the Navy annex | -3416 ft | 180 ft | 32504 ft |
| implausibly ground-hugging | -3416 ft | 120 ft | 48681 ft |
To simplify our notation, let θ(t) = t v0 / r where v0 is the constant air speed and r is the radius as calculated from the formula above (and shown in the table for each of the three flight paths). θ(t) will be the angle in radians subtended by the arc that extends from the origin to f(t). Then the circular solution for our new boundary value problem is
f(t) = r ‹ sin (θ(t)), 1 - cos (θ(t)) ›
Using the chain rule to calculate first and second derivatives, we get
f ′(t) = v0 ‹ cos (θ(t)), sin (θ(t)) ›
f ″(t) = v02 / r ‹ - sin (θ(t)), cos (θ(t)) ›
The equation for the first derivative says the scalar velocity (air speed) is always v0, although its directional components depend upon the time t. The equation for the second derivative says the scalar acceleration is always v02 / r, although its directional components depend upon the time t. Since we will have to add 1 g of vertical acceleration for earth's gravity, the maximal g-load occurs when the acceleration is entirely vertical, at the origin.
The following table shows the scalar accelerations and g-loads for each of our three flight paths. The scalar accelerations are very slightly less than the decelerations we calculated for the parabolic solutions, because both the air speed and ground speed are slightly less for most of the flight path, but the only difference that remains visible after rounding to three significant digits is the scalar acceleration for the flight path that goes over the VDOT antenna.
| flight path | scalar acceleration | g-load |
|---|---|---|
| over the VDOT antenna | 28.2 ft/sec2 | 1.9 g |
| over the Navy annex | 18.8 ft/sec2 | 1.6 g |
| implausibly ground-hugging | 12.5 ft/sec2 | 1.4 g |
At the resolution used earlier to display the parabolic approach, the tiny differences between parabolic and circular approaches are invisible.
The reason we have obtained such similar results from the parabolic and circular solutions is that the difference in elevation between the Navy annex and the Pentagon is small compared to the horizontal distance between the two, which means the angle subtended by the circular arc is fairly small. For small angles near the origin, there isn't much difference between a circular arc and a parabolic segment that approximates that circular arc. For larger angles, the difference is greater; that's why we see a difference (in the third decimal place) for the flight path over the VDOT antenna but not for flight paths with lesser changes in elevation.
Given the large uncertainty in the flight path's height near the VDOT antenna or Navy annex, the tiny difference in acceleration between a parabolic solution and a circular solution is unimportant.
We now leave the realm of legitimate mathematics and return to Rob Balsamo's video.
Of course we came under harsh criticism for such a mistake. Some critics used formulas which is based on a one-dimensional problem such as a car decelerating over a distance. This formula is completely incompatible with the two-dimensional problem we are addressing.
-- Rob Balsamo, 9/11: Attack on the Pentagon, 3:15-3:38
As shown above, the correct solution to the 2-dimensional problem coincides with the correct solution to the 1-dimensional problem.
This was pointed out several times, but it appears the critics would rather plug their ears.
-- Rob Balsamo, 9/11: Attack on the Pentagon, 3:51-4:16
Balsamo complains about his critics because he can't do the math.
As I have demonstrated above, there exist several flight paths for which the g-load is well within the capability of a Boeing 757. Those flight paths demonstrate that all of Balsamo's assertions to the contrary are wrong.
A parabola formula was also offered in response to our article. [....] Unfortunately for this scenario, it wasn't consistent with the original premise of our article, or the data provided by the government.
-- Rob Balsamo, 9/11: Attack on the Pentagon, 4:18-4:35
The 1-dimensional calculation is entirely consistent with the 2-dimensional calculation. Both of those calculations imply a parabolic flight path, and the parabolic flight path is almost indistinguishable from a circular arc whose constant air speed is the same as the parabolic path's constant ground speed.
After about eight minutes of mathematical double talk designed to give the false impression that Balsamo actually knows something about mathematics and physics, he resorts to a classic bait-and-switch: Instead of proving that all possible flight paths would imply impossible g-loads, he argues for one particular flight path based on his individual interpretation of the flight data recorder (whose own positional data indicate that its data end before the VDOT antenna is even reached) and of the NTSB animation (which even the NTSB disclaims). Balsamo then attempts to show that his own favorite flight path is impossible.
Yes, Balsamo's favorite interpretation of the data is impossible. That proves nothing about more plausible interpretations of the data.
Placing the aircraft on the south path, lowered...to the top of the VDOT antenna, we can examine the pullup needed at pole 1 and measure the radius using a 3-point arc radius tool provided with this 3-D animation software program.
-- Rob Balsamo, 9/11: Attack on the Pentagon, 8:52-9:13
Balsamo has drawn his own favorite flight path to scale. Any measurements or calculations based on that drawing will apply only to Balsamo's favorite flight path. They will not apply to more plausible flight paths.
Without giving any reason, Balsamo assumes his favorite flight path is well approximated by a circular arc. His software won't tell him any different, so long as the three points he picks aren't all in a straight line, because any three non-collinear points lie on some circle. Balsamo should have checked his assumption by adding a fourth point. If that fourth point lies on or near the circle, then the circle may be a good approximation. If the fourth point does not lie on or near the circle, however, then the circle is a bad approximation and any calculations based on it will be misleading. Apparently Balsamo did not perform that basic check.
Given the video's resolution, I cannot see Balsamo's favorite flight path clearly enough to discern its shape in the region of his three points. With a piecewise linear flight path, with a distinct bend where two linear pieces are joined, a calculating practitioner can come up with an arbitrarily small radius by placing one of his three points at the bend and the other two on opposite sides of the bend, as close as necessary to achieve the desired radius. Hence curve-fitting on curves of dubious provenance is an inherently suspect enterprise.
Balsamo came up with a radius of 2085 feet, which is less than 10% of the radius I calculated for a genuinely circular arc that connects the top of the VDOT antenna to level flight at the base of the Pentagon. My calculations are correct, so Balsamo either made a mistake in his measurement, or chose a flight path whose acceleration is ten times the acceleration required for a genuinely circular arc.
After adding 1 g for earth's gravity, Balsamo gets a total of 10.14 g. As shown above, the correct value for a circular arc that passes above the VDOT antenna and ends in level flight at the base of the Pentagon is 1.9 g. Balsamo's "corrected" calculation is off by a factor of 5.
9/11: Attack on the Pentagon combines meaningless technobabble with hand-waving and curve-fitting to one short segment of a specific flight path that has been carefully selected for its implausibility.
The generalization from that one flight path to all possible flight paths is completely unjustified. It is easy to describe plausible flight paths whose g-loads are well within the capabilities of a Boeing 757, and I have done so in this review.
The video amounts to an argument from authority. The self-assuredness of Balsamo's video monologue, combined with his sneering contempt for critics, is designed to convince viewers that he really does understand the physics and mathematics, while his detractors do not. In reality, Rob Balsamo is a former airline pilot with no discernible skills in mathematics or physics and a history of making outrageous mistakes in his calculations.
If you approach his video with an open mind, however, then it should occur to you to wonder what people who are fluent in calculus and Newtonian mechanics think of its arguments.
Now you know.
William D Clinger, PhD
(MIT, 1981, mathematics)
First posted 24 September 2009. Last updated 25 September 2009 (cosmetic editing).